Skip to Main Content
免費試用

      

Ansys 部落格

January 23, 2024

有限差分時域法 (FDTD) 是什麼？

有限差分時域法 (FDTD) 是一種 3D 全波電磁求解器，常用於為奈米光子裝置、製程和材料建模。

儘管在光子學領域，FDTD已成為行業標準，但有限元素（FEM）和動差法（MoM）則是高頻電子學中主要的標準計算電磁求解器，各自都有其優勢。本文將特別著重於光子模擬的 FDTD。

FDTD 是由 Kane S. Yee 於 1966 年首次提出的演算法，以解決 James Clerk Maxwell 的轉型方程式 (正式名稱為 Maxwell 方程式)。這些方程式構思於19 世紀，不只整合了電力與磁力，還為無線電、電視和無線通訊等技術奠定了基礎。1980 年代以前，Yee 的數值法並未被廣泛稱為 FDTD。

Maxwell 的 FDTD 方程式

Maxwell 方程式及相關定律包括：

  • Gauss 電場定律：說明電荷如何產生電場
  • Gauss 磁場定律：說明磁場如何不具有孤立磁極
  • Faraday 電磁感應定律：解釋變化的磁場如何在電路中感生電磁力 (EMF)
  • Ampere 定律加上 Maxwell 新增的發現：連接電流與磁場，整合變化電場的作用。
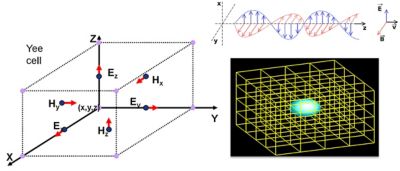
FDTD method

在 FDTD 中，每個場域元件都會在網格單元 (Yee 網格) 內略有不同的位置解決，如上所示。

FDTD 的運作方式為何？

在 FDTD 中，模擬域是由模擬區域截斷，並由網格離散化的空間。當 FDTD 模擬執行時，電磁 (EM) 場會從每個網格單元的 Maxwell 方程式計算得出，且解決方案會重複進行時間步級。空間離散化可呈現複雜的幾何和結構，而時間離散化則會隨著時間擷取 EM 場的演進。

FDTD 有哪些應用？

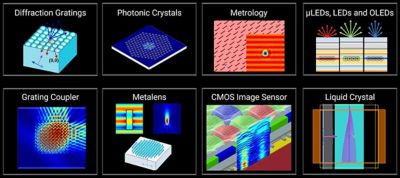
FDTD 方法通常適用於某些或全部的物體尺寸與光波長大小相當的設計案例。FDTD 的準確度和多功能性使其成為各種光子設計的必備求解器，包括：

雖然 FDTD 提出了最常用的 Maxwell 方程式解決方案，但針對特定應用中有其他更有效率的方法，像是包含了多層光學膜和繞射式光學元件的設計。同樣地，採用光子整合元件的求解器組合策略也可以更有效地處理不同結構，例如採用特徵模態展開 (EME) 方法來達到導光結構。建立一套針對正確問題選擇正確求解器的策略性方法，可能會影響設計程序速度與效率，以及結果的準確度。 

FDTD method nanophotonics

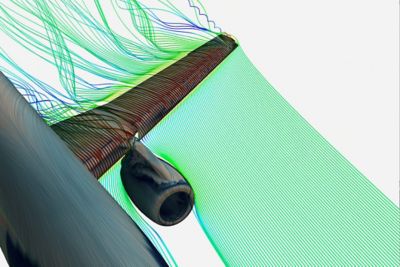
FDTD 方法廣泛用於奈米光子設計。

FDTD 的優點為何？

設計師可以利用 FDTD，徹底研究不同材質和結構對光線極化與波長相依的相互作用，取得關於光學現象的深入資訊，例如反射率、透射、繞射、干涉和吸收。

  • 時域和頻域分析：FDTD 提供 EM 場隨時間演進的動態視圖。時域解決方案的內建自動化 Fourier 轉換，讓頻率分析變得更簡單。 
  • 寬頻功能：由於 FDTD 是一種時域方法，因此能在單一模擬中更快地計算寬頻結果。
  • 複雜的幾何形狀：FDTD 擅長複雜幾何建模，可以處理任意形狀的結構。
  • 準確度和多功能性：此方法本質上即無任何實體近似值，因此使其具備高多功能性與準確度。

FDTD 有哪些挑戰？

FDTD 方法的高準確度和多功能性帶來一些挑戰，包括：

  • 模擬尺寸：能夠準確建模的裝置最大實體尺寸，最終受限於可用的運算資源。
  • 模擬時間：FDTD 模擬的速度取決於多項因素，從模擬設定和體積到運算系統的硬體規格。在 3D 模式中，模擬時間預期會隨著下列關係成比例變化：~V (l/dx)4，其中 V 代表模擬體積，dx 為網格大小。因此，網格準確度和設計大小會大幅影響模擬時間。
  • 記憶體與運算能力：FDTD 模擬中的大量空間與時間未知數，會隨著更精細的網格與更大型的模擬體積呈指數增長，進而產生極大量的記憶體和運算能力需求。
  • 記憶體頻寬：處理器之間的記憶體存取和大量資料交換，特別是處理大量模擬時，是加速 FDTD 模擬的主要挑戰。

如何加速 FDTD 模擬？

Ansys Lumerical 採用多種進階方式來加速 FDTD 模擬。

微調演算法

數十年來，Lumerical 的 FDTD 演算法已在基礎上經過微調，將運算額外負擔降至最低，同時提供最高準確度。有多項專利和進階功能可協助簡化模擬設定，包括網格、監控器、來源、結構、材料、分析群組等。內建先進的最佳化架構，可額外加速產生最佳化的奈米光子裝置。  

平行運算

Ansys Lumerical FDTD 具有高度最佳化的運算引擎，可利用多核心 CPU 運算系統，並在高效能運算 (HPC) 叢集中運用圖形處理器 (GPU) 的平行架構。CPU 和 GPU 架構在平行處理方面均表現出色，可解決 FDTD 模擬中同時運算的需求。HPC 系統運用這個平行處理來分散工作負載，大幅提升模擬效能。大型模擬工作可分割為多個獨立的運算執行緒，並可在數小時內平行執行超過 500-1000 億個網格單元的大型模擬作業。

隨著模擬的複雜度增加，對運算資源效率與可擴充性的需求也隨之增加。這就是雲端運算與 HPC 的動態組合發揮作用之處，革新 FDTD 模擬。Lumerical 解決方案提供與 CPU 和 GPU 相容的模擬軟體，可讓使用者部署在內部部署或雲端環境。

如需更多關於 HPC 和雲端上 FDTD 模擬軟體的資訊，請觀看我們「利用 HPC 和雲端加速光子設計」的網路研討會。

若要深入瞭解基礎的求解器物理學及如何設定、執行和分析 FDTD 模擬，請參閱 Ansys 創新課程中的 FDTD 學習課程。